How many moles of electron weigh one kilogram

 4.5/5

Want create site? Find Free WordPress Themes and plugins.

How many moles of electron weigh one kilogram To determine how many moles of electrons weigh one kilogram, we start by acknowledging the mass of a single electron, which is approximately

9.109 \times 10^{-31}

kilograms. Given one kilogram equals

1, 000

grams, and

6.022 \times 10^{23}

electrons make up one mole, the calculation begins by determining how many electrons are in one kilogram. This calculates to about

1.097 \times 10^{30}

electrons. To convert this into moles, divide by Avogadro’s number, resulting in approximately

1.824 \times 10^{6}

moles. This shows the incredibly small individual electron mass and underscores the vast scale of Avogadro’s number in connecting atomic-scale quantities to the macroscopic measure of kilograms

Understanding the Mass of an Electron
Calculating the Number of Moles in One Kilogram
The Relationship Between Mass and Moles in Chemistry
Conclusion
FAQs

Understanding the Mass of an Electron

Understanding the Mass of an Electron

Fundamental Particle: An electron is a fundamental subatomic particle with a negative charge that orbits the nucleus of an atom. It is one of the building blocks of matter, essential for the structure and behavior of atoms and molecules.
Mass of an Electron: The mass of a single electron is incredibly small, approximately $9.109 \times 10^{-31}$ kilograms. Despite its small size, electrons play a crucial role in determining the chemical and physical properties of elements.
Measurement Significance: Understanding the mass of electrons is fundamental in various scientific disciplines, from quantum mechanics to chemistry and materials science. It influences calculations involving atomic structure, particle interactions, and the behavior of electrons in electric and magnetic fields.
Relative Scale: Compared to other subatomic particles like protons and neutrons, electrons have significantly less mass. This distinction affects how electrons interact with each other and with atomic nuclei, influencing atomic bonding and the properties of matter.
Experimental Determination: The precise measurement of electron mass has been refined through experiments using techniques such as mass spectrometry and other advanced spectroscopic methods, contributing to our understanding of atomic and molecular physics.

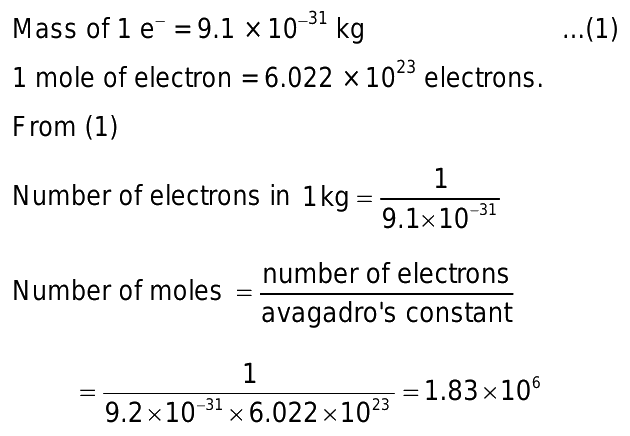
Calculating the Number of Moles in One Kilogram

Understanding Molar Quantity: In chemistry, a mole represents a specific quantity of atoms, molecules, or particles. One mole contains Avogadro’s number ( $6.022 \times 10^{23}$ ) of entities.
Using Mass Conversion: To calculate the number of moles in one kilogram of a substance, you first determine the substance’s molar mass (in grams per mole). For electrons, knowing their individual mass ( $9.109 \times 10^{-31}$ kilograms), the calculation involves converting kilograms to grams and then using Avogadro’s number.
Applying the Formula: The formula to calculate moles from mass is:
$particles\text{Number of moles} = \frac{\text{Mass in kilograms}}{\text{Molar mass in kilograms}} \times \frac{1 \text{ mole}}{6.022 \times 10^{23} \text{ particles}}$
For electrons, the mass is divided by their individual mass in kilograms to find the total number.
Example Calculation: For electrons, given their mass and Avogadro’s number, one kilogram of electrons translates to approximately $1.824 \times 10^6$ moles.
Significance in Chemistry: This calculation is fundamental in stoichiometry, determining reactant quantities, and understanding the scale of particles in macroscopic terms, pivotal in chemical reactions and industrial processes.

The Relationship Between Mass and Moles in Chemistry

The relationship between mass and moles in chemistry is crucial for understanding and quantifying the amount of substances involved in chemical reactions and processes.

Definition of Moles: A mole is a unit used to count entities, such as atoms, molecules, or ions. One mole of any substance contains Avogadro’s number ( $6.022 \times 10^{23}$ ) of those entities.
Molar Mass: Molar mass is the mass of one mole of a substance, expressed in grams per mole (g/mol). It is numerically equal to the atomic or molecular weight of the substance.
Calculating Moles from Mass: To determine the number of moles in a given mass of a substance, divide the mass (in grams) by the molar mass (in g/mol):
$g/mol)\text{Number of moles} = \frac{\text{Mass (in grams)}}{\text{Molar mass (in g/mol)}}$
Converting Between Mass and Moles: This relationship allows chemists to convert between mass and moles and vice versa, enabling precise measurements and calculations in chemical reactions, stoichiometry, and formulation of solutions.
Application in Stoichiometry: In chemical equations, the ratio of moles of reactants and products determines their proportions. This fundamental concept governs how substances interact and transform during chemical reactions.

Conclusion

In conclusion, How many moles of electron weigh one kilogram the relationship between mass and moles in chemistry is fundamental to understanding and quantifying the amount of substances involved in chemical reactions and processes. Moles provide a way to count atoms, molecules, or ions on a macroscopic scale, with one mole containing Avogadro’s number of entities. The concept of molar mass connects the microscopic scale of individual particles to the macroscopic scale of grams per mole, facilitating conversions between mass and moles. This relationship is essential in stoichiometry, where the proportions of reactants and products in chemical reactions are determined based on moles. Overall, grasping this relationship enables chemists to predict outcomes, formulate solutions, and conduct precise measurements, underscoring its importance across various scientific disciplines and practical applications in chemistry.

FAQs

Q: 1What is the mass of a single electron?

Ans: The mass of a single electron is approximately $9.109 \times 10^{-31}$ kilograms.

Q:2 How do you calculate the number of electrons in one kilogram?

Ans: To calculate the number of electrons in one kilogram, divide the mass of one kilogram by the mass of a single electron ( $9.109 \times 10^{-31}$ kg/electron). This yields approximately $1.097 \times 10^{30}$ electrons.

Q:3 Why is Avogadro's number important in this calculation?

Ans Avogadro’s number ( $6.022 \times 10^{23}$ electrons/mol) is crucial because it relates the number of particles (electrons, in this case) to moles. It allows us to convert the number of electrons into moles.

Q: 4How many moles of electrons are there in one kilogram?

Ans: One kilogram contains approximately $1.824 \times 10^{6}$ moles of electrons. This calculation involves dividing the number of electrons in one kilogram by Avogadro’s number.

Did you find apk for android? You can find new Free Android Games and apps.